by Stefan Hollos

There are many different types of bonds but most can be classified into two broad categories: coupon bonds and zero coupon bonds, also known as discount bonds. The following discussion will concentrate on coupon bonds which we will refer to as just bonds from here on. We will say a little about zero coupon bonds at the end.

In its most basic form, a bond is a loan that pays simple interest. The amount of the loan is called the face value of the bond 1 and the interest payments are called coupon payments 2. The coupon payments are fixed amounts that correspond to a fixed percentage of the face value 3 . This percentage is called the coupon yield.

The coupon payments are made periodically. The usual period is six months but it may also be just once a year. The payments are made until the maturity date of the bond. At maturity, the face value of the bond is payed.

You can think of a bond as a lease on an amount of money equal to the face value. The coupon payments are the rent on the money and the lease expires on the maturity date when the money is returned.

To analyze bonds in greater detail we need to define some variables.

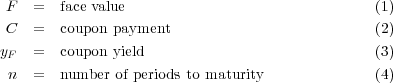
F = face value (1) C = coupon payment (2) yF = coupon yield (3) n = number of periods to maturity (4)

Now let’s assume that you simply collect all the coupon payments over the life of the bond and the face value at maturity. Your total cash flow from the bond will then be

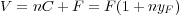V = nC + F = F (1+ nyF)
(5)

This is what you would expect from a simple interest investment.

It is also possible, at least in principle, to turn a bond into a compound interest investment. If each coupon payment is reinvested at the rate yF and compounded at the same intervals as the payments then the bond becomes a compound interest investment. To see how this works, look at the sum of all the cash flows in this case.

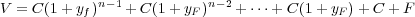n-1 n-2 V = C(1+ yf) + C (1 + yF) + ⋅⋅⋅+ C(1+ yF)+ C + F
(6)

The sum is a geometric series that can be collapsed to give

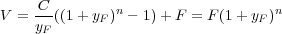-C- n n V = yF ((1 +yF ) - 1)+ F = F(1+ yF )
(7)

This is a compound interest investment of F at the rate yF for n periods.

Whether or not the coupond payments can actually be reinvested like this is another question. It is unlikely that each coupon can be reinvested at yF over the remaining life of the bond. Interest rates fluctuate constantly so that the coupons may end up being invested at rates higher or lower than yF. This brings us to our next question: what happens to the value of a bond when the interest rates change?

When a bond is first issued, a coupon yield is selected such that investors will be willing to pay for the bond at face value. The current risk free interest rate, the credit worthiness of the company, and inflation expectations are some of the factors that determine an attractive coupon yield. If these factors remain unchange then the value of the bond will remain unchanged at its face value.

Suppose however that the risk free interest rate drops so that new bonds, comparable to the old ones, are selling with lower coupon yields. This will make the older bonds, with the higher coupon yields, more valuable. If the older bonds are sold then they will have a market value greater than the face value.

Likewise, if the interest rates go up then the market value of the old bonds will be less than the face value. It is therefore possible to realize capital gains and losses on a bond if it is sold when the interest rates are different from when it was bought.

If P is the current market price for a bond then one measure of the return from the bond is the current yield which is defined as

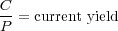C- = current yield P
(8)

If the price is greater than the face value then the current yield will be less than the coupon yield and vice versa.

Current yield is not a good measure of return because it does not take into account the payment of the face value at maturity, which can result in a capital gain or loss. The most widely used measure of bond yield is called the yield to maturity which we will denote as yM. To find yM you equate the remaining cash flows from the bond, invested at yM, with the price of the bond invested at yM. This gives the following equation:

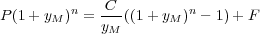P (1+ yM )n = -C-((1 + yM)n - 1) +F yM
(9)

This equation needs to be solved numerically for yM. A calculator for yM can be found here.

The yield to maturity is important since it is the measure that is most commonly used to compare bonds.

Zero coupon bonds are much simpler to analyze since there is only a single cash flow which is the payment of the face value at maturity. This of course means that the bond must sell at a discount to its face value. These bonds usually have short maturity times and there are no coupon payments to reinvest.